How To Calculate Keq

  1. How To Calculate Keq From Concentrations
  2. How To Calculate Keq Of A Reaction

Voiceover Our goal is to calculate the equilibrium constant K for this reaction, so for this reaction right here. Now we're gonna use the standard reduction potentials to do so. So in the previous video, we talked about the relationship between the equilibrium constant K and the standard cell potential. A variation of Type 1 problems is when you are given the Keq and all the equilibrium concentrations except one and you are asked to calculate that one. The solution for this type of problem is simply writing out the Keq expression, filling in what you know and solving for the unknown. Read through this example: At 200°C, the Keq for the.

Calculating Equilibrium Constants

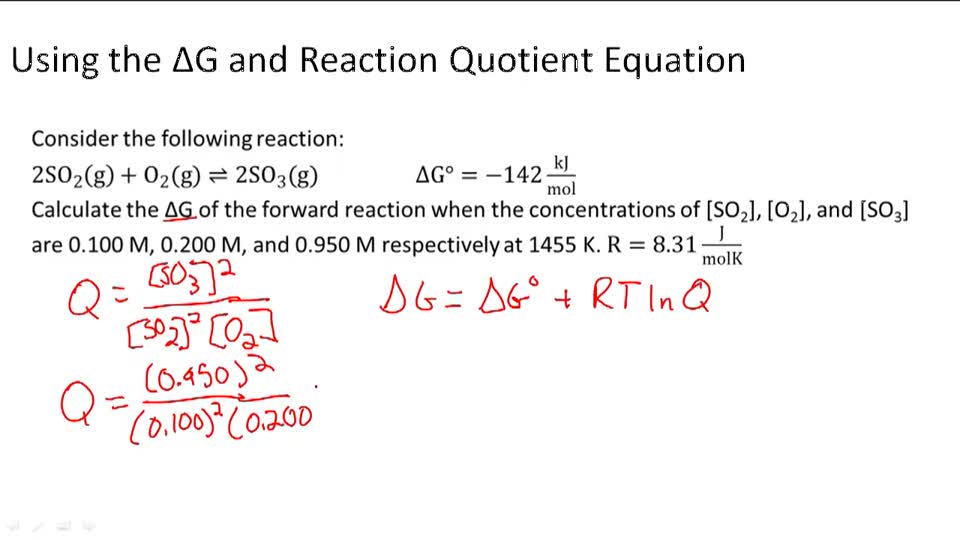
We need to know two things in order to calculate the numeric value of the equilibrium constant:

  • the balanced equation for the reaction system, including the physical states of each species. From this the equilibrium expression for calculating Kc or Kp is derived.
  • the equilibrium concentrations or pressures of each species that occurs in the equilibrium expression, or enough information to determine them. These values are substitued into the equilibrium expression and the value of the equilibrium constant is then calculated.
Calculating K from Known Equilibrium Amounts
  • Write the equilibrium expression for the reaction.
  • Determine the molar concentrations or partial pressures of each species involved.
  • Subsititute into the equilibrium expression and solve for K.
Example: Calculate the value of the equilibrium constant, Kc , for the system shown, if 0.1908 moles of CO2, 0.0908 moles of H2, 0.0092 moles of CO, and 0.0092 moles of H2O vapor were present in a 2.00 L reaction vessel were present at equilibrium.

CO + H2O' height =34 src='EquilibriumArt/CalcKexample1.gif' width=288 NOSAVE>

  • Write the equlibrium expression for the reaction system.
Calculate
  • Since Kc is being determined, check to see if the given equilibrium amounts are expressed in moles per liter (molarity). In this example they are not; conversion of each is requried.


    [CO2] = 0.1908 mol CO2/2.00 L = 0.0954 M
    [H2] = 0.0454 M
    [CO] = 0.0046 M
    [H2O] = 0.0046 M

  • Substitute each concentration into the equilibrium expression and calculate the value of the equilibrium constant.

Calculating K from Initial Amounts and One Known Equilibrium Amount

  • Write the equilibrium expression for the reaction.
  • Determine the molar concentrations or partial pressures of each species involved.
  • Determine all equilibrium concentrations or partial pressures using an ICE chart.
  • Substitute into the equilibrium expression and solve for K
Example: Initially, a mixture of 0.100 M NO, 0.050 M H2, 0.100 M H2 O was allowed to reach equilibrium (initially there was no N2 ). At equilibrium the concentration of NO was found to be 0.062 M. Determine the value of the equilibrium constant, Kc , for the reaction:

N2 + 2 H2O' height=32 src='EquilibriumArt/CalcKexample2.gif' width=326 >

  • Write the equilibrium expression for the reaction.
  • Check to see if the amounts are expressed in moles per liter (molarity) since Kc is being . In this example they are.
  • Create an ICE chart that expresses the initial concentration, the change in concentration, and the equilibrium concentration for each species in the reaction. From the chart you can determine the changes in the concentrations of each species and the equilibrium concentrations. From the example, we start with the folowing information.
NO
H2
N2
H2O
Initial Concentration (M)
0.100
0.0500
0
0.100
Change in Concentration (M)

- 2 x

- 2 x

+ x

+ 2 x

Equilibrium Concentration (M)
0.062

The change in concentration of the NO was (0.062 M - 0.100M) = - 0.038 M. Thus -2 x = - 0.038 and x = 0.019. Note: the negative sign indicates a decreasing concentration, not a negative concentration. The changes in the other species must agree with the stoichiometry dictated by the balance equation. The hydrogen will also change by - 0.038 M, while the nitrogen will increase by + 0.019 M and the water will increase by + 0.038 M. From these changes we can complete the chart to find the equilibrium concentrations for each species.

NO
H2
N2
H2O
Initial Concentration (M)
0.100
0.0500
0
0.100
Change in Concentration (M)
- 0.038
- 0.038
+ 0.019
+ 0.038
Equilibrium Concentration (M)
0.062
0.012
0.019
0.138
  • Substitute the equilibrium concentrations into the equilibrium expression and solve for Kc.

Calculating K from Known Initial Amounts and the Known Change in Amount of One of the Species

  • Write the equilibrium expression for the reaction.
  • Determine the molar concentrations or partial pressures of each species involved.
  • Determine all equilibrium concentrations or partial pressures using an ICE chart.
  • Substitute into the equilibrium expression and solve for K.
Example: A flask is charged with 3.00 atm of dinitrogen tetroxide gas and 2.00 atm of nitrogen dioxide gas at 25oC and allowed to reach equilibrium. It was found that the pressure of the nitrogen dioxide decreased by 0.952 atm. Estimate the value of Kp for this system:

2 NO2' height =29 src='EquilibriumArt/CalcKexample3.gif' width=181 NOSAVE>

  • Write the equilibrium expression to find Kp.
p expression' height =60 src='EquilibriumArt/Kpexpression.gif' width=122 NOSAVE>
  • Check to see that the given amounts are measured in appropriate pressure units since Kp is to be . In this example they are (atmospheres).
  • Create an ICE chart and calculate the changes in pressure and equilibrium pressures for each species.
N2O4
NO2
Initial Pressure (atm)
3.00
2.00
Change in Pressure (atm)
+ 0.476
- 0.952
Equilibrium Pressure (atm)
3.476
1.048
  • Substitute the equilibrium pressures into the expression for Kp and solve for Kp.
p' height =53 src ='EquilibriumArt/Kpsolution.gif' width =210 NOSAVE >

A chemical system can be thought of as being either:

1.At equilibrium

2.Not at equilibrium

A system which is not at equilibrium will move spontaneously to a position of being at equilibrium.

What is Keq : The 'K' in Keq stands for 'Constant'. The 'eq' means that the reaction is at equilibrium. Very roughly, Keq tells you the ratio of Products/Reactants for a given reaction at equilibrium at a certain temperature.

K eq = [Products] / [Reactants]

Consider the reaction

How To Calculate Keq From Concentrations

2HI(g ) < ---------------> H2(g) + I2(g)

that if you take the [H2], the [I2] and the [HI] in an equilibrium mixture of these at 423 °C,the expression

[H 2 ] [I 2 ] / 2 [HI] = 0.0183

this is Keq expression this is value of Keq

How

Writing Keq Expressions:

Consider the general reaction:

aA + bB <------> cC + dD

where, A,B,C,D denote the reactants and prodcuts and a,b,c,d are coefficients in the balanced chemical equation.The equilibrium-constant expression for a reaction is an expression obtained by multiplying the concentrations of products,dividing by th concentration of reactants and raising each concentration term to a power equal to the coefficent in the chemical equation.

The equilibrium constant Kc is the value obtained for the equilibrium-constant expression when equilibrium concentrations are substituted.

Keq= [C]c [D]d / [A]a [B] b

Here you denote the molar concentration of a substance by writing its formula is square brackets.The subscript eq on the equilibrium contant means that it is defined in terms of molar concentrations.

The Keq Expressions for Solids and Liquids

For solids: When we write the Keq expression for a reaction with solids, we simply leave out the solids.

the Keq expression for the equation:

CaCO3(s) <---------> CaO(s) яАл + CO2(g)

is simply: Keq = [CO2]

The same argument that was used for solids can also be used for liquids. Thus, we can expand the last statement:

How To Calculate Keq Of A Reaction

'When we write the Keq expression for a reaction with solids or liquids, we simply leave out the solids and the liquids'.

Gases and aqueous solutions do undergo changes in concentration so they are always

included in the Keq expression.